لماذا خلق الله العين كرية الشكل

بؤرة العدسة الكرية تقع علي سطحها و للتأكد من ذلك أحضر كرة زجاجية و ضعها ملاصقة لعين الناظر ثم أستخدم عدسة محدبة عادية لامة بحيث تكون بؤرتها تقع علي سطح العدسة الكرية (عدسة كرة) و بذلك يمكنك أن تري ما هو مقابل لتلك العدسة اللامة و يعملان معا كمقراب(منظار مكبر) و بذلك تكون تلك التجربة كدليل علي أن بؤرة العدسة الكرية تقع علي سطحها . تطبيقات علي هذه العدسة : 1-عدسة العين كرية الشكل و سبحان من خلق الشبكية ملاصقة لسطح كرة العين و تعمل كحائل لأستقبال الصور عليها . 2- نحن نري الكون من داخل عدسة كرية سوف تخرج الشمس من مغربها عند كشط السماء كما قال العليم الكريم في سورة التكوير عندما تكشط السماء سوف تكور الأرض و تخرج من مغربها . 3 - حركة الصور للمشاهد من داخل العدسة الكرية علي سطحها عكس الحركة الفعلية للجسم خارج العدسة . 4- صدق الله العظيم إذا يقول (و سخر لكم الشمس و القمر دائبين و سخر لكم اليل و النهار ) و من النظر للآية الكريمة يقرن الله الليل بالشمس و يقرن القمر بالنهار و ذلك من الاستدلال من العاطف و المعطوف علية من سياق الأية الكريمة و بمقارنة تلك الأية الكريمة مع الأيات رقم (30-40) سورة العنكبوت نجد ان الله لم يحدث أختلاف بين ذكر أسماء الأقوام و العذاب الذي وقع بهم في سورة العنكبوت و مع العلم أن ربي لا يضل و لا ينسي فلماذا إذا يقرن الله الشمس بالليل و القمر بالنهار و لكنها تلك هي الحقيقة أننا نري الكون من داخل عدسة كرية تظهر صور الأشياء خارجها علي سطحها في الجزء العكسي من العدسة و للتأكد من ذلك راجع رسم أشعة فان ألن التي توضح تمدد الأينوسفير وقت النهار و هو في الجهة العكسية من الشمس و بذلك حقت كلمة رب العالمين الذي قال و له أختلاف الليل و النهار . منقول عن عصام عبد الوكيل

زاوية الاسناد والنسب المثلثية

زاوية الاسناد

هي الزاوية الحادة المحصورة بين محور السينات علي المستوي الديكارتي وبين ضلع الانتهاء للزاوية .

الحالة الأولي  

اذا كانت الزاوية تقع في الربع الثاني فالزاوية المكافئة علي الصورة ( 180 - هـ ) حيث أن هـ زاوية الاسناد .

وتكون النسب المثلثية كالأتي 

جتا ( 180 - هـ ) = - س = - جتا هـ


جا ( 180 - هـ ) = ص = جا هـ 


ظا ( 180 - هـ ) = - ص / س = - ظا هـ 


مثال / أوجد قيمة النسب المثلثية الأتية 


أ - جتا 1110


1110 درجة تكافئ ( 1110 - ( 3 * 360 )) = 30 درجة


جتا 1110 = - جتا 30 = - 0.86


نكمل لاحقا الحالة الثانية والثالثة ..

تحديد اشارة الاقترانات المثلثية للزاوية في الوضع القياسي

1- اذا كان ضلع الانتهاء للزاوية يقع في الربع الاول فان كلا من الاحداثي  س وص موجبه ( + ) وبالتالي جميع الاقترانات ومقلوبها موجبه .


2- اذا كان ضلع الانتهاء يقع في الربع الثاني  فان س > 0 وص < 0 وعلي ذلك فان الجيب ومقلوبها فقط موجب والباقي سالب .


3- اذا وقع ضلع الانتهاء للزاوية في الربع الثالث فان كل من س و ص سالبة وبالتالي فان الظل ومقلوبها فقط موجب والباقي سالب .


4- اذا وقع ضلع الانتهاء للزاوية في الربع الرابع فان جيب التمام ومقلوبها فقط موجبات والباقي سالب .  

المجال المغناطيسي لتيار يمر في ملف دائري

التجربة العملية 

الغرض من التجربة :


تخطيط المجال المغناطيسي لتيار يمر في ملف دائري 

الأدوات :


 ورق مقوى – بوصلات – سلك – أسلاك توصيل – أميتر – مفتاح – بطارية – برادة حديد – ريوستات 

خطوات العمل : 

1- نصل دائرة كما بالرسم 

2- نضع البوصلة قرب أحد جانبي اللفة ثم نغلق الدائرة ونحرك البوصلة حول اللفة
 ونلاحظ اتجاه القطب الشمالي للبوصلة 

3- ننقل البوصلة إلى الطرف الآخر للفة ونكرر ما سبق 

4- نفتح الدائرة ونعكس قطبي البطارية ثم نقفل الدائرة 

5- ونكرر الخطوات السابقة وندون الاستنتاج 

6- نفتح الدائرة ونبعد البوصلة وننثر برادة الحديد على جانبي
اللفة وعند المركز نغلق الدائرة ونطرق بلطف على لوح الورق المقوى ونلاحظ البوصلات 

شكل المجال 

تفقد خطوط المجال دائريتها حول كل من فرعي الملف ، وتكون خطوط المجال عبارة عن دوائر بيضاوية تتزاحم داخل الملف وتتباعد خارجه وعند المحور تكاد تكون مستقيمة 

تحديد نوع القطب في كل من فرعي الملف : 

الوجه الذي يمر فيه التيار في اتجاه عقارب الساعة ( قطب جنوبي ) 

الوجه الذي يمر فيه التيار في عكس عقارب الساعة ( قطب شمالي 

Average vs. Instantaneous Speed

During a typical trip to school, your car will undergo a series of changes in its speed. If you were to inspect the speedometer readings at regular intervals, you would notice that it changes often. The speedometer of a car reveals information about the instantaneous speed of your car. It shows your speed at a particular instant in time.

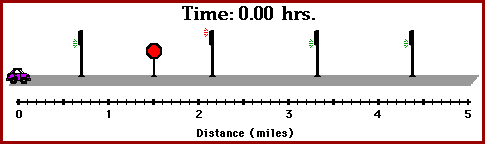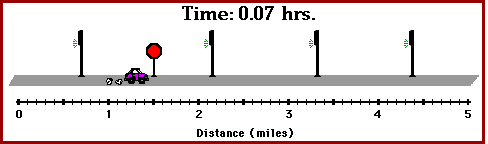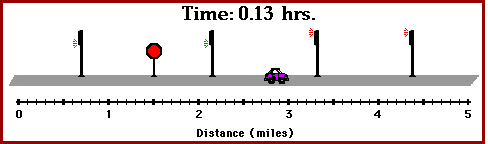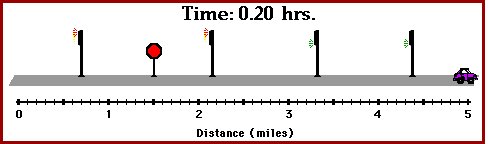
The instantaneous speed of an object is not to be confused with the average speed. Average speed is a measure of the distance traveled in a given period of time; it is sometimes referred to as the distance per time ratio. Suppose that during your trip to school, you traveled a distance of 5 miles and the trip lasted 0.2 hours (12 minutes). The average speed of your car could be determined as

On the average, your car was moving with a speed of 25 miles per hour. During your trip, there may have been times that you were stopped and other times that your speedometer was reading 50 miles per hour. Yet, on average, you were moving with a speed of 25 miles per hour

Newton's First Law

Newton's First Law

 the variety of ways by which motion can be described (words, graphs, diagrams, numbers, etc.) was discussed. In this unit (Newton's Laws of Motion), the ways in which motion can be explained will be discussed. Isaac Newton (a 17th century scientist) put forth a variety of laws that explain why objects move (or don't move) as they do. These three laws have become known as Newton's     three laws of motion. The focus of Lesson 1 is Newton's first law of motion

 sometimes referred to as the law of inertia

Newton's first law of motion is often stated as

An object at rest stays at rest and an object in motion stays in motion with the same speed and in the same direction unless acted upon by an unbalanced force

 

There are two parts to this statement - one that predicts the behavior of stationary objects and the other that predicts the behavior of moving objects. The two parts are summarized in the following diagram

The behavior of all objects can be described by saying that objects tend to "keep on doing what they're doing" (unless acted upon by an unbalanced force). If at rest, they will continue in this same state of rest. If in motion with an eastward velocity of 5 m/s, they will continue in this same state of motion (5 m/s, East). If in motion with a leftward velocity of 2 m/s, they will continue in this same state of motion (2 m/s, left). The state of motion of an object is maintained as long as the object is not acted upon by an unbalanced force. All objects resist changes in their state of motion - they tend to "keep on doing what they're doing

Suppose that you filled a baking dish to the rim with water and walked around an oval track making an attempt to complete a lap in the least amount of time. The water would have a tendency to spill from the container during specific locations on the track. In general the water spilled when:

• the container was at rest and you attempted to move it
• the container was in motion and you attempted to stop it
• the container was moving in one direction and you attempted to change its direction.

The water spills whenever the state of motion of the container is changed. The water resisted this change in its own state of motion. The water tended to "keep on doing what it was doing." The container was moved from rest to a high speed at the starting line; the water remained at rest and spilled onto the table. The container was stopped near the finish line; the water kept moving and spilled over container's leading edge. The container was forced to move in a different direction to make it around a curve; the water kept moving in the same direction and spilled over its edge. The behavior of the water during the lap around the track can be explained by Newton's first law of motion.

Everyday Applications of Newton's First Law

There are many applications of Newton's first law of motion. Consider some of your experiences in an automobile. Have you ever observed the behavior of coffee in a coffee cup filled to the rim while starting a car from rest or while bringing a car to rest from a state of motion? Coffee "keeps on doing what it is doing." When you accelerate a car from rest, the road provides an unbalanced force on the spinning wheels to push the car forward; yet the coffee (that was at rest) wants to stay at rest. While the car accelerates forward, the coffee remains in the same position; subsequently, the car accelerates out from under the coffee and the coffee spills in your lap. On the other hand, when braking from a state of motion the coffee continues forward with the same speed and in the same direction, ultimately hitting the windshield or the dash. Coffee in motion stays in motion.

Have you ever experienced inertia (resisting changes in your state of motion) in an automobile while it is braking to a stop? The force of the road on the locked wheels provides the unbalanced force to change the car's state of motion, yet there is no unbalanced force to change your own state of motion. Thus, you continue in motion, sliding along the seat in forward motion. A person in motion stays in motion with the same speed and in the same direction ... unless acted upon by the unbalanced force of a seat belt. Yes! Seat belts are used to provide safety for passengers whose motion is governed by Newton's laws. The seat belt provides the unbalanced force that brings you from a state of motion to a state of rest. Perhaps you could speculate what would occur when no seat belt is used.

There are many more applications of Newton's first law of motion. Several applications are listed below. Perhaps you could think about the law of inertia and provide explanations for each application.




Blood rushes from your head to your feet while quickly stopping when riding on a descending
elevator. 
The head of a hammer can be tightened onto the wooden handle by banging the bottom of the handle 


against a hard surface. 
A brick is painlessly broken over the hand of a physics teacher by slamming it with a hammer.

الزوايا وقياسها

الزاوية هي عبارة عن اتحاد شعاعين في نقطة تسمي رأس الزاوية ومن الممكن ان يكون قياس الزاوية موجبا ومن الممكن ان يكون قياسها سالبا .


اذا كان الدوران مع عقارب الساعة يكون القياس سالبا اما اذا كان الدوران بعكس عقارب الساعة يكون القياس موجبا .


الزاوية لها ضلعان هما ضلع الابتداء الذي يبدأ من عنده الدوران وضلع الانتهاء الذي ينتهي من عنده الدوران وهو الذي يحدد موقع الزاوية في ارباع المستوي .

الزاوية القياسية التي يقع فيها ضلع الانتهاء علي أحد المحوريين الاحداثيين هي زاوية ربعية .